Pedro Nunes, Mercator and Escher

In the sixteenth century, the royal cosmographer Pedro Nunes discovered the rhumb line and explained that the shortest distance to be travelled by a ship between two points on the Earth is not a straight line but an arc. Escher represented this idea 300 years later.

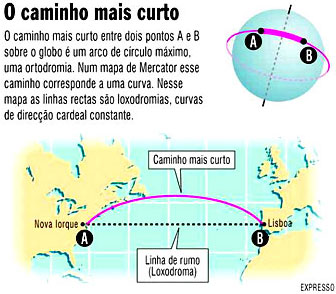
Whoever travels from Lisbon to New York normally approaches the North American coast at least an hour before arriving at the destination. During that hour, the traveller observes the coastline of the State of Massachusetts, the island of Martha’s Vineyard and other tourist destinations. The aeroplane then flies over Long Island and its beaches, travelling from North to South in direction of the city of New York. Looking at the map, it seems as if the pilot made a huge detour and that instead of flying straight over the Atlantic, he preferred to find the New World to the North, where the Portuguese approached it for the first time, and only then descend to his destination.

On looking at a globe, however, one realises that one travelled along the shortest route possible, which corresponds to a great circle arc, i.e. the arc of a circle that contains the starting point and the destination and which has its centre in the centre of the Earth. If a piece of string is stretched over the globe with one end situated over Lisbon and the other over New York, it is clear to see that the shortest distance does in fact follow the coast of Massachusetts. This means that, in order to follow the shortest route between two points lying on a very similar latitude – 39 degrees for Lisbon and 41 degrees for New York –, the aeroplane starts by flying eastwards and a little to the North and ends up travelling along westwards and a little to the South.

All this may seem simple and obvious, yet it took a long time for the navigators to understand. The first person to fully understand it was Pedro Nunes (1502-1579).

The royal cosmographer was in fact confronted with the inverse problem, which is a more difficult one: in order to travel between two points, is it advisable to always travel in the same direction? The problem was suggested to him by Martim Afonso de Sousa, founder of Portugal’s first colonies in Brazil. Wishing to travel from Rio de Prata to Lisbon, he realised that travelling westwards was not enough and that he needed to also travel northwards. More importantly, it was not easy to understand the precise direction in which he should travel.

Pedro Nunes’ genius lies in clearly distinguishing two possible routes for a ship in deep sea. One would be the shortest distance between the two points, corresponding to a great circle arc – the so-called great circle route. Another would be the route followed by a ship that followed a constant direction in relation to the cardinal points – the so-called rhumb line, later known as loxodrome. Only in very special cases do the two routes coincide: when one is travelling along the equator, from East to West or from West to East, or when one travels along one of the meridians, from North to South or from South to North. In all other cases, the two routes do not coincide. In order to travel the shortest route between Lisbon and New York, aeroplanes do not follow a rhumb line; they constantly change their cardinal direction so as to be able to follow a great circle route.

On discovering the rhumb line, Pedro Nunes also proved that a boat which followed the same cardinal direction in a planet completely covered with water, hypothetically, would not end up at the same starting point, as thought at the time, but that it would follow an infinite spiral, called a loxoromic spiral, getting closer and closer to one of the poles but only reaching it after an infinite number of rounds.

The most spectacular drawings of loxodromic spirals are, without a doubt, those of Mauritus Cornelius Escher (1898-1972). In 1958, Escher created some drawings of spheres with spirals, seemingly without realising the profound historical and geometrical meaning. The drawing represented here is called the “Bolspiralen”, spherical spirals, and is one of the most beautiful examples. It is a wooden engraving in four blocks, each one having its own colour, with a diameter of 32 cm. We can imagine a boat that decides to constantly follow a rhumb line at an angle of approximately 60 degrees in a North-South direction. The spirals show the route followed by the boat and also shows that, irrespective of its starting point, its course will converge. The spirals are wider close to the equator and become narrower as they near the poles.

Besides showing navigators the routes followed when a constant cardinal direction is followed, Pedro Nunes’ loxodromics had a decisive effect on our vision of the world. They determined the manner in which maps began to be made and created the vision we have of the continents today. Our mathematician received the intuition that the ancient Portolano maps had to be remade but it was Gerardus Mercator (1512-1594) who would bring about a revolution in cartography, based on the discovery of Pedro Nunes.

The major problem of creating flat maps is the appearance of inevitable distortions. In the same way as an orange peel breaks and becomes deformed when pressed down onto a table, so map makers have to distort the geometry of the globe in order to be able to reproduce a spherical surface on a flat surface. The distortions are not serious when the areas involved are reduced. However, the world had become greater with the journeys of the Discoveries and maps of continents and oceans, vital for navigators, were starting to be made.

Mercator, who knew the results of Pedro Nunes, decided to create a map that would directly serve navigators. As a first principle, he decided that the map would have a net, in which the lines of equal latitude would all be parallel to the equator and perpendicular to the meridians, and in which the meridians would all be parallel among themselves. As a second principle, Mercator decided that the rhumb lines would appear as straight lines, whereby he progressively increased the distances between the parallels as the latitude approached the poles. Thus the so-called Mercator projection was born, which is still the best known and most used today.

The Mercator map is what is called a conformal map, as it preserves the direction between any two points on the globe. However the Mercator map introduces inevitable distortions, which moulded our geographical intuition. In this map, Greenland seems enormous, bigger than South America, when in fact the latter has an area nines times that of the former. The map also deceives modern travellers, which are still amazed with the routes followed by aeroplanes.


Nuno Crato