|
 |
|
 |
|
|
|
|
|
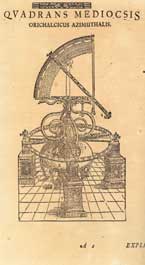
Quadrant with a radius of 390 mm, in which the nonius of Pedro Nunes is integrated, as shown in the illustration presented in Tycho Brahe’s Astronomiae Instauratae Mechanica, ed 1602.
|
|
Quadrant with a radius of 580 mm, in which the nonius of Pedro Nunes and the diagonal scale are integrated, as shown in the illustration presented in Tycho Brahe’s Astronomiae Instauratae Mechanica, ed 1602.
|
|
|
|
|
|
 |
|
 |
|
|
|
|
|
Portrait of Tycho Brahe in Astronomiae Instauratae Mechanica, ed. 1602. Biblioteca da Ajuda, Lisbon.
|
|
Mural quadrant in Tycho Brahe’s Astronomiae Instauratae Mechanica, ed. 1602. Biblioteca da Ajuda, Lisbon.
|
|
|
|
|
|
Tycho Brahe turns to the nonius of Pedro Nunes
Following these considerations, we might even go so far as to say that Pedro Nunes never possessed a single specimen of the nonius he designed.
Until recently, there were only two quadrants known that made use of the nonius. These are referred by Tycho Brahe (1546-1601), in his work Astronomiae Instauratae Mechanica, the first edition of which dates back to 1598. Besides the illustrations of the referred quadrants presented, in this work the author states – and we are basing ourselves on the English translation of 1946 – that they are divided with the usual transversals, yet also make use of the nonius of the “famous Spanish mathematician”. The translation seems to us to be incorrect as the term effectively used by Brahe is “hispanicus”, which we believe includes all the inhabitants of the Iberian Peninsula and not just the Spanish, in particular. In addition, the Danish astronomer states that Pedro Nunes ascribes the method to Ptolemy, which does not really convince him, however. In our opinion, he is paying homage to the modesty of our great mathematician.
The reason that led Tycho Brahe to use the nonius was his preoccupation in using great precision in his astronomical observations. This induced him to lay down important concepts, which won converts and gave rise to a new Astronomy. Only in this way was it possible to draw up the journals of celestial phenomena with absolute precision. For this reason, this astronomer takes a place of honour in the History of Astronomy.
We know that Brahe manufactured his own instruments, possibly with the help of assistants. In the twenty-year period in which he had his observatory on the island of Ven, 27 kilometres from Copenhagen, an observatory which had been given to him by the king of Denmark Frederic II, he built 20 instruments, none of which survived, as is the case with all the others he produced. We thus lost an opportunity of seeing Nunes’ invention included in the work of the greatest astronomer of his time.
The nonius was not used by Brahe for a long time. In a letter addressed to Cristopher Rothmann dated 20 January 1587, the famous Danish astronomer writes: “However, as soon as I started trying to measure the exact altitude of the celestial bodies with the help of quadrants, I realised from the experience that the common division, taken as far as possible, was not enough in the small instruments. I therefore turned to the subtle process which Nunes presents in the third postulate of his De Crepusculis and made it more exact, increasing the number of subdivisions and calculating tables by which it would be possible to immediately and accurately know the altitude of any point. And as this invention of Nunes, just as experience had proven to me, did not live up to his promises, I ask myself if the process used to divide a straight line into very small parts by means of transversal points could not be applied to curved lines as well”.
This letter brings us some new facts. First of all, it tells us that Tycho increased the number of positions of the nonius, which an analysis of the illustrations presented in Astronomiae Instauratae Mechanic does not reveal. We are willing to admit that this experiment might have been performed on a large quadrant of which we were not aware.
|
 |
|
|
|
(click on the picture
above to see an enlarged version)
Nonius used for azimuthal readings. Drawing from the manuscript Jewell of Artes by George Waymouth, 1604. British Library, London.
|
|
|
Secondly, it tells us that Brahe drew up tables to calculate the angles corresponding to the numerous positions of the nonius, which must have been arduous work, which leads us to believe that his calculation was done for one or more nonius using more positions than those designed by its inventor. Further on, we will see that Clavius calculated tables for a new version of the nonius.
With regard to the abandonment of the nonius, already mentioned by Tycho Brahe in the text transcribed above, we have a further piece of information from Delambre, dating from the beginning of the nineteenth century, that affirms that Tycho renounced its use, “which he would not have done had the errors not been greater than one or two minutes”.
The most important theoretical use of the nonius is owed to captain George Waymouth who, in his manuscript The Jewell of Artes, presents several quadrants for use in astronomy and artillery. And it is precisely in this work that for the first time there is mention of the nonius being used in the evaluation of horizontal angles.
In our country, nothing of this ever happened. Besides the nautical astrolabe, which was developed and manufactured by the Portuguese, albeit using elementary technology, and which at the time and beyond any doubt was the most prestigious instrument for measuring altitudes, there is no recollection of a planispheric astrolabe, a vital instrument for the cosmographers of yore, ever having been made in Portugal. Of these beautiful instruments, over 1300 reached our days, as the list that refers this number dates back to 1955, and since then many others have been catalogued.
|
|